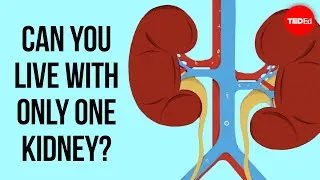
Dubbelklik op de Engelse ondertitels hieronder om de video af te spelen.
New videos
Deze site laat u kennismaken met YouTube-video's die nuttig zijn om Engels te leren. U ziet Engelse lessen gegeven door topdocenten uit de hele wereld. Dubbelklik op de Engelse ondertitels op elke videopagina om de video af te spelen. De ondertitels scrollen synchroon met het afspelen van de video. Heeft u opmerkingen of verzoeken, neem dan contact met ons op via dit contactformulier.